Home » Subject » Algebra

Non-separable center of a lattice (Solved)

Importance: Medium ✭✭
Author(s): Porton, Victor
Subject: Algebra
Keywords: lattice
Recomm. for undergrads: no
Posted by: porton
on: September 11th, 2008
Solved by:

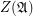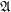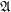I will call center $ Z(\mathfrak{A}) $ of a bounded distributive lattice $ \mathfrak{A} $ the sublattice of all complemented elements of $ \mathfrak{A} $.

I will call a bounded distributive lattice 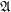$ \mathfrak{A} $ a lattice with separable center when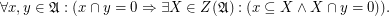 $$\forall x,y\in\mathfrak{A}: (x\cap y=0\Rightarrow \exists X\in Z(\mathfrak{A}):(x\subseteq X\wedge X\cap y = 0)) .$$

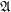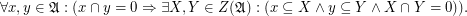
Equivalently a bounded distributive lattice with separable center is such a bounded distributive lattice $ \mathfrak{A} $ that $$\forall x,y\in\mathfrak{A}:(x\cap y=0\Rightarrow\exists X,Y\in Z(\mathfrak{A}):(x\subseteq X\wedge y\subseteq Y\wedge X\cap Y = 0)) .$$

Conjecture   There exist bounded distributive lattices which are not with separable center.

(Previously this problem was erroneously stated without the word distributive, it is corrected now.)

This conjecture follows from William Elliot's post in sci.math and the criterion of a lattice to be distributive, see here.

So the problem solves positively.

Admins: Should I delete this problem from Open Problem Garden because its solution was too easy (found in less than one day). Or just to leave it marked as solved?

Bibliography



* indicates original appearance(s) of problem.
add new comment