 login/create account
login/create account
Enumerating the number of binary relations
Problem
Consider the finite set ![$ [n]=\{1,\dots,n\} $](/files/tex/5e73d98d00a2a88ad490e4ca6382ad3cc0177bc9.png) . We define a binary relation
. We define a binary relation  over the set
over the set ![$ [n] $](/files/tex/06d9f8dd53868a1233f15c435c46e03deb17a82a.png) and we write
and we write 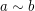 to indicate
to indicate  is related to
is related to 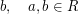 . We have the following properties of
. We have the following properties of  :
:
(i) 
(ii)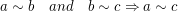
A relation  together with a set
together with a set ![$ [n] $](/files/tex/06d9f8dd53868a1233f15c435c46e03deb17a82a.png) is a set of unordered pairs denoted by
is a set of unordered pairs denoted by ![$ ([n],R) $](/files/tex/6f426e00823dbeca041586e699a9aeccf52e0b4a.png) . Consider the example
. Consider the example ![$ ([n],R_1)=\{(1,2),(2,3),(4,12),(6,7)\} $](/files/tex/32b774d199829010006ffe06ee870f3a60e5ac5f.png) . This is extendable to
. This is extendable to ![$ ([n],R_2)=\{(1,2),(2,3),(1,3),(4,12),(6,7)\} $](/files/tex/de417f419b99e482ab4b72e307426319335c964d.png) . In this case we call
. In this case we call 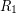 to be equivalent to
to be equivalent to 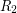 (over
(over ![$ [n] $](/files/tex/06d9f8dd53868a1233f15c435c46e03deb17a82a.png) ).(This defines a equivalence relation over the set of relations) Two relations are called distinct if they are not equivalent.
).(This defines a equivalence relation over the set of relations) Two relations are called distinct if they are not equivalent.
Let  be all the equivalent relations. A set
be all the equivalent relations. A set ![$ ([n],R_i) \quad \forall 1\leq i\leq k $](/files/tex/0f265286df50c7093eb7a4675b0b51d5b5f9be9c.png) is said to be a minimal relation iff there does not exist a relation
is said to be a minimal relation iff there does not exist a relation 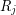 which is extendable to
which is extendable to 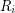 .
.
1.Do all minimum relations among  have same cardinality ie., same number of pairs?
have same cardinality ie., same number of pairs?
2.Find the number of distinct relations
(i) when points are numbered
(ii) Upto isomorphism.
ps: I am a newcomer to this garden and newcomer to research too. Admin please move to relevant section if problem is not appropriate for this section.
Bibliography
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University