See Wikipedia's growth of groups for definitions of the basics reguarding growth rate in groups (in particular polynomial and exponential growth rates). A finitely generated group has intermediate growth if its growth rate (for every finite generating set) is subexponential but superpolynomial.

Most naturally occuring groups have either polynomial growth (such as 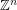) or exponential growth (such as a free group with rank 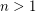). Milnor [M] famously asked if there exists a finitely generated group with intermediate growth, and this problem was resolved in the affirmative by Grigorchuk [G]. The groups constructed by Grigorchuk are not finitely presented, thus leaving the above problem.

Expert opinion seems to be that there are no finitely presented groups of intermediate growth.