 login/create account
login/create account
inward reloid
Funcoidal products inside an inward reloid ★★
Author(s): Porton
Conjecture (solved) If 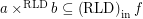 then
then 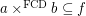 for every funcoid
for every funcoid  and atomic f.o.
and atomic f.o.  and
and  on the source and destination of
on the source and destination of  correspondingly.
correspondingly.
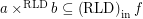 then
then 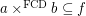 for every funcoid
for every funcoid  and atomic f.o.
and atomic f.o.  and
and  on the source and destination of
on the source and destination of  correspondingly.
correspondingly. A stronger conjecture:
Conjecture If 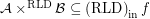 then
then 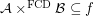 for every funcoid
for every funcoid  and
and 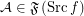 ,
, 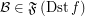 .
.
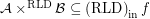 then
then 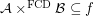 for every funcoid
for every funcoid  and
and 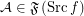 ,
, 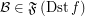 .
. Keywords: inward reloid
Distributivity of inward reloid over composition of funcoids ★★
Author(s): Porton
Keywords: distributive; distributivity; funcoid; functor; inward reloid; reloid
Reloid corresponding to funcoid is between outward and inward reloid ★★
Author(s): Porton
Keywords: funcoid; inward reloid; outward reloid; reloid
Inward reloid corresponding to a funcoid corresponding to convex reloid ★★
Author(s): Porton
Keywords: convex reloid; funcoid; functor; inward reloid; reloid
Funcoid corresponding to inward reloid ★★
Author(s): Porton
Keywords: funcoid; inward reloid; reloid

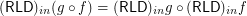 for any composable
for any composable 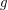 .
. ![\[ ( \mathsf{\tmop{RLD}})_{\tmop{out}} f \subseteq g \subseteq ( \mathsf{\tmop{RLD}})_{\tmop{in}} f \Leftrightarrow ( \mathsf{\tmop{FCD}}) g = f. \]](/files/tex/def032a20d7d57db67d77c0f002f1c9f2909fe98.png)
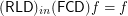 for any
for any 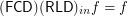 for any
for any  Drupal
Drupal CSI of Charles University
CSI of Charles University