Home » Subject » Combinatorics
Importance: Medium ✭✭
Author(s): Landman, Bruce M.
Robertson, Aaron
Subject: Combinatorics
Keywords: Fibonacci numbers
monochromatic diffsequences
Recomm. for undergrads: no
Posted by: vjungic
on: August 24th, 2008
Question   Is the set of Fibonacci numbers 3-accessible?

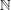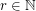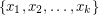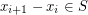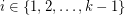A set $ S $ is $ r $-accessible if for any $ r $-coloring of $ \mathbb{N} $, $ r\in \mathbb{N} $, there exist long monochromatic $ S $-diffsequences, i.e., for any $ k\in \mathbb{N}\backslash \{ 1\} $ there is a monochromatic sequence $ \{ x_1,x_2,\ldots ,x_k\} $ such that $ x_{i+1}-x_i \in S $ , for all $ i\in \{ 1,2,\ldots ,k-1\} $.

The set of Fibonacci numbers 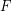$ F $ is 2-accessible. [LR1]

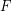$ F $ is not 6-accessible. [AGJL]

It is known that a 3-coloring of any 27 consecutive positive integers yields a monochromatic 4-term 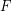$ F $-diffsequence.

Bibliography

[AGJL] Hayri Ardal, David Gunderson, Veselin Jungi\'c, and Bruce Landman, {\it On Accessibility of the Set of Fibonacci Numbers}, In Preparation

*[LR1] Bruce M. Landman and Aaron Robertson, {\it Avoiding Monochromatic Sequences With special Gaps}, SIAM J. Discrete Math Vol. 21 (2007), no. 3, 794--801.

[LR2] Bruce M. Landman and Aaron Robertson, Ramsey Theory on the Integers, Stud. Math. Libr. 24, AMS Providence, RI, 2004


* indicates original appearance(s) of problem.
add new comment

Reply

Comments are limited to a maximum of 1000 characters.
More information about formatting options