 login/create account
login/create account
Conjecture Let 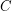 be a circuit in a bridgeless cubic graph
be a circuit in a bridgeless cubic graph  . Then there is a five cycle double cover of
. Then there is a five cycle double cover of  such that
such that 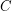 is a subgraph of one of these five cycles.
is a subgraph of one of these five cycles.
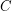 be a circuit in a bridgeless cubic graph
be a circuit in a bridgeless cubic graph  . Then there is a five cycle double cover of
. Then there is a five cycle double cover of  such that
such that 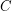 is a subgraph of one of these five cycles.
is a subgraph of one of these five cycles. A cycle in  is meant to be a
is meant to be a 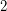 -regular subgraph of
-regular subgraph of  . A five cycle double cover of
. A five cycle double cover of  is a set of five cycles of
is a set of five cycles of  such that every edge of
such that every edge of  is contained in exactly two of these cycles.
is contained in exactly two of these cycles.
This conjecture is a combination and thus strengthening of the  -cycle double cover conjecture and the strong cycle double cover conjecture.
-cycle double cover conjecture and the strong cycle double cover conjecture.
Bibliography
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University