 login/create account
login/create account
For  let
let 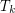 denote the minimal number
denote the minimal number  such that there is a rainbow
such that there is a rainbow 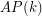 in every equinumerous
in every equinumerous  -coloring of
-coloring of 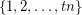 for every
for every 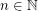
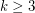 ,
, 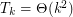 .
. A  -coloring of
-coloring of 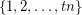 is equinumerous if each color is used
is equinumerous if each color is used  times. An arithmetic progression is rainbow if it does not containt two terms of the same color.
times. An arithmetic progression is rainbow if it does not containt two terms of the same color.
In [JLMNR] it was proved that 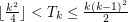 .
.
It is known that 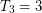 ([AF], [JR]) and
([AF], [JR]) and 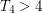 ([CJR]). It is not hard to show that
([CJR]). It is not hard to show that 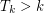 for all
for all 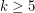 ([AF]).
([AF]).
Bibliography
[AF] Maria Axenovich, Dmitri Fon-Der-Flaass: On rainbow arithmetic progressions, Electronic Journal of Combinatorics, 11, (2004), R1.
[CJR] David Conlon, Veselin Jungic, Rados Radoicic, On the existence of rainbow 4-term arithmetic progressions, Graphs and Combinatorics, 23 (2007), 249-254
*[JLMNR] Veselin Jungic, Jacob Licht (Fox), Mohammad Mahdian, Jaroslav Nesetril, Rados Radoicic : Rainbow arithmetic progressions and anti-Ramsey results, Combinatorics, Probability, and Computing - Special Issue on Ramsey Theory, 12, (2003), 599--620.
[JNR] Veselin Jungic, Jaroslav Nesetril, Rados Radoicic: Rainbow Ramsey theory, Integers, The Electronic Journal of Combinatorial Number Theory, Proceedings of the Integers Conference 2003 in Honor of Tom Brown, 5(2), (2005), A9.
[JR] Veselin Jungic, Rados Radoicic : Rainbow 3-term arithmetic progressions, Integers, The Electronic Journal of Combinatorial Number Theory, 3, (2003), A18.
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University