 login/create account
login/create account
Elementary symmetric of a sum of matrices
Problem
Given a Matrix  , the
, the  -th elementary symmetric function of
-th elementary symmetric function of  , namely
, namely 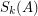 , is defined as the sum of all
, is defined as the sum of all  -by-
-by- principal minors.
principal minors.
Find a closed expression for the  -th elementary symmetric function of a sum of N
-th elementary symmetric function of a sum of N  -by-
-by- matrices, with
matrices, with 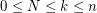 by using partitions.
by using partitions.
The Newton-Girard formulas imply particular expressions for small values of  and
and 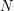 , for example,
, for example,  .
.
Bibliography
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University
that's ok
that's ok