 login/create account
login/create account
Equality in a matroidal circumference bound
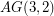 the only 3-connected matroid for which equality holds in the bound
the only 3-connected matroid for which equality holds in the bound 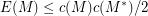 where
where 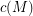 is the circumference (i.e. largest circuit size) of
is the circumference (i.e. largest circuit size) of  ?
? If  is a 2-connected matroid with at least two elements then it was proved in [LO] that
is a 2-connected matroid with at least two elements then it was proved in [LO] that 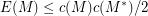 where
where 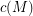 is the size of the largest circuit in
is the size of the largest circuit in  .
.
Equality can hold in this bound -- in particular the binary affine cube 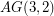 is an 8-element self-dual matroid with circumference 4. There are various graphic matroids for which equality holds, and these have been classified in [W] where it is shown that they are all series-parallel networks and hence not 3-connected.
is an 8-element self-dual matroid with circumference 4. There are various graphic matroids for which equality holds, and these have been classified in [W] where it is shown that they are all series-parallel networks and hence not 3-connected.
This question is therefore asking whether 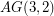 is the sole
is the sole  -connected example where equality holds; this is known to be true for all matroids on up to 9 elements.
-connected example where equality holds; this is known to be true for all matroids on up to 9 elements.
(A variant of this question would be to ask if 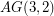 is the only non-graphic example other than trivial modifications like replacing every element with an equally sized parallel class.)
is the only non-graphic example other than trivial modifications like replacing every element with an equally sized parallel class.)
Bibliography
[LO] Lemos, Manoel; Oxley, James A sharp bound on the size of a connected matroid. Trans. Amer. Math. Soc. 353 (2001), no. 10, 4039--4056 MathSciNet
[W] Wu, Pou-Lin Extremal graphs with prescribed circumference and cocircumference. Discrete Math. 223 (2000), no. 1-3, 299--308 MathSciNet
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University