 login/create account
login/create account
Partition of Complete Geometric Graph into Plane Trees
For a set  of
of  points in the plane with no three collinear, the complete geometric graph
points in the plane with no three collinear, the complete geometric graph  has vertex set
has vertex set  and edge set consisting of the
and edge set consisting of the 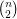 straight line-segments between each pair of points in
straight line-segments between each pair of points in  .
.
Since each subtree of  has at most
has at most 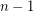 edges, every partition of
edges, every partition of 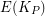 into subtrees has at least
into subtrees has at least 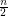 parts. The conjecture asks for such a partition into exactly
parts. The conjecture asks for such a partition into exactly 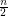 subtrees, such that in addition, no two edges in each subtree cross.
subtrees, such that in addition, no two edges in each subtree cross.
It is folklore that the conjecture is true if  is in convex partition. In fact, the edge set of the complete convex graph can be partitioned into plane Hamiltonian paths. Bose et al. [BHRW] characterised all possible partitions of the complete convex graph into plane spanning trees. Bose et al. [BHRW] also proved that every complete geometric graph on
is in convex partition. In fact, the edge set of the complete convex graph can be partitioned into plane Hamiltonian paths. Bose et al. [BHRW] characterised all possible partitions of the complete convex graph into plane spanning trees. Bose et al. [BHRW] also proved that every complete geometric graph on  vertices can be partitioned into at most
vertices can be partitioned into at most 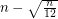 plane subtrees.
plane subtrees.
I heard about this conjecture from Ferran Hurtado in 2003, but the problem is much older than that.
Bibliography
[BHRW] Prosenjit Bose, Ferran Hurtado, Eduardo Rivera-Campo, David R. Wood. Partitions of complete geometric graphs into plane trees, Computational Geometry: Theory & Applications 34(2):116-125, 2006. MathSciNet
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University
This conjecture is false
This conjecture has recently been disproved, see arXiv:2108.05159 and arXiv:2112.08456.