Home » Subject » Topology

Which compact boundaryless 3-manifolds embed smoothly in the 4-sphere?

Importance: High ✭✭✭
Author(s): Kirby
Subject: Topology
Keywords: 3-manifold
4-sphere
embedding
Recomm. for undergrads: no
Posted by: rybu
on: November 7th, 2009
Problem   Determine a computable set of invariants that allow one to determine, given a compact boundaryless 3-manifold, whether or not it embeds smoothly in the 4-sphere. This should include a constructive procedure to find an embedding if the manifold is embeddable.

For general 3-manifolds this problem is fairly wide-open. But for some specific families of 3-manifolds it is heavily investigated.

There are two common embedding constructions: (1) obtain your 3-manifold as 0-surgery on a link which is the disjoint union of two smooth slice links. (2) Obtain your 3-manifold as the boundary of a Mazur manifold -- where Mazur manifold is taken to be a contractible 4-manifold constructed as 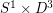$ S^1 \times D^3 $ union a 2-handle. In both cases the resulting 3-manifold M embeds smoothly in 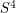$ S^4 $. There are many other embedding constructions but no known "uniform" construction that works for all embeddable 3-manifolds.

Since such a 3-manifold would bound two 4-manifolds on either side, the embedding problem is a type of double cobordism problem, and related to issues such as the problem of determining which homology 3-spheres bound homology 4-balls.

The smoothness in the assumption is important. Mike Freedman has proven all homology 3-spheres admit tame topological embeddings into 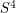$ S^4 $. These embeddings have a less combinatorial nature than smooth embeddings so it is somewhat natural to restrict to the question of smooth embeddings. For example, the Poincare Homology Sphere does not embed smoothly in 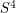$ S^4 $, since it has a non-trivial Rochlin invariant.

Related problems

Which homology 3-spheres bound homology 4-balls?

Bibliography

[B] R. Budney, Embeddings of 3-manifolds in the 4-sphere from the point of view of the $ 11 $-tetrahedron census, arXiv preprint arXiv:0810.2346

[CH] J.S. Crisp, J.A. Hillman, Embedding Seifert fibred $ 3 $-manifolds and $ {\rm Sol</em>\sp 3 $-manifolds in $ 4 $-space,} Proc. London Math Soc. (3) (1998), no. {\bf 3} 685--710.

[KK] A.~Kawauchi, S.~Kojima, Algebraic classification of linking pairings on $ 3 $-manifolds, Math. Ann. {\bf 253} (1980), no. 1, 29--42.

[FS] R.~Fintushel, R.~Stern, Rational homology cobordisms of spherical space forms, Topology, {\bf 26} no. 3 pp. 385--393, (1987).

[GL] P.M.~Gilmer, C.~Livingston, On embedding 3-manifolds in 4-space, Topology, {\bf 22}, no. 3, pp. 241--252 (1983).

*[K] Kirby, R. Problem list in low-dimensional topology. [http://math.berkeley.edu/~kirby/problems.ps.gz]

[L] R.A.~Litherland, Deforming twist-spun knots, Trans. Amer. Math. Soc. {\bf 250} (1979), 311--331.


* indicates original appearance(s) of problem.
add new comment