 login/create account
login/create account
A construction on boolean lattices is itself a boolean lattice? (Solved)
Let 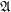 and
and 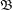 be (fixed) boolean lattices (with lattice operations denoted
be (fixed) boolean lattices (with lattice operations denoted  and
and  , bottom element
, bottom element 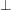 and top element
and top element 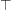 ).
).
I call a boolean funcoid a pair 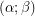 of functions
of functions 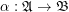 ,
, 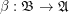 such that (for every
such that (for every 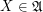 ,
, 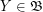 )
) 
(Boolean funcoids are a special case of pointfree funcoids as defined in my free ebook.)
Order boolean funcoids by the formula 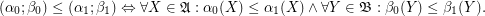
If this conjecture does not hold in general, does it hold for: a. atomic boolean lattices? b. atomistic boolean lattices? c. complete boolean lattices?
For the special case when 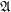 and
and 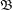 are complete atomic boolean lattices, the conjecture easily follows from this math.SE answer.
are complete atomic boolean lattices, the conjecture easily follows from this math.SE answer.
See Algebraic General Topology for definitions of used concepts.
It is mostly solved:
It is not a complete answer, but the most important cases are considered. So I mark this question as solved. Further consideration however is welcome.
Bibliography
*First appeared as this math.SE question.
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University
A special case proved
I have proved a weird (due its asymmetry) special case of this conjecture:
Theorem. The set of pointfree funcoids between a complete boolean lattice and an atomistic boolean lattice is itself a boolean lattice.
See my blog.
Victor Porton - http://www.mathematics21.org