 login/create account
login/create account
Forcing a $K_6$-minor
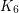 -minor.
-minor. 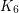 -minor.
-minor. The first conjecture implies the second.
Whether the second conjecture is true was first asked in [KT]. Both conjectures were stated in [BJW].
The second conjecture is implied by Jørgensen’s conjecture, which asserts that every  -connected
-connected 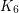 -minor-free graph is apex (which have minimum degree at most
-minor-free graph is apex (which have minimum degree at most  and are thus not
and are thus not 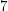 -connected). Since Jørgensen’s conjecture is true for sufficiently large graphs [KNTWa,KNTWb], the second conjecture is true for sufficiently large graphs.
-connected). Since Jørgensen’s conjecture is true for sufficiently large graphs [KNTWa,KNTWb], the second conjecture is true for sufficiently large graphs.
Bibliography
*[BJW] János Barát, Gwenaël Joret, David R. Wood. Disproof of the List Hadwiger Conjecture, Electronic J. Combinatorics 18:P232, 2011.
*[KT] Ken-ichi Kawarabayashi and Bjarne Toft. Any 7-chromatic graph has 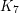 or
or 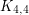 as a minor. Combinatorica 25 (3), 327–353, 2005.
as a minor. Combinatorica 25 (3), 327–353, 2005.
[KNTWa] Ken-ichi Kawarabayashi, Serguei Norine, Robin Thomas, Paul Wollan. 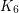 minors in
minors in  -connected graphs of bounded tree-width. http://arxiv.org/abs/1203.2171
-connected graphs of bounded tree-width. http://arxiv.org/abs/1203.2171
[KNTWb] Ken-ichi Kawarabayashi, Serguei Norine, Robin Thomas, Paul Wollan. 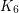 minors in large
minors in large  -connected graphs. http://arxiv.org/abs/1203.2192
-connected graphs. http://arxiv.org/abs/1203.2192
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University