 login/create account
login/create account
Inscribed Square Problem
A Jordan curve is a continuous function  from the closed interval
from the closed interval ![$ [0,1] $](/files/tex/f65c588a56e7eca31976481db4eeeae4e72a4d3a.png) to the plane
to the plane 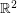 with the properties that
with the properties that  is injective on the half-open interval
is injective on the half-open interval 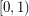 (i.e.,
(i.e.,  is simple) and
is simple) and 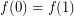 (i.e.,
(i.e.,  is closed).
is closed).
Bibliography
[M] Meyerson, M.D., Equilateral triangles and continuous curves, Fund. Math. 110, (1980), 1--9.
* indicates original appearance(s) of problem.
inscription of squares in simple closed curves
There is a theorem that says:
in all simple closed curves there are 4n points that are vertex of n squares (inf = > n > =1)
Jorge Pasin.
in all simple closed curves there are 4n points
Would you clarify? An obtuse triangle has only one inscribed square, so this theorem is not true for n>=2. Do you have a reference to this theorem? Strashimir Popvassilev
Is the conjecture known to
Is the conjecture known to be true for C^1-smooth curves?
no.
If it were true for C^1 curves, then since a Jordan curve is compact, it may be weierstrass approximated by a series of C^1 curves (indeed by curves whose component functions are polynomials) such that the series converges uniformly to the given jordan curve. Then by assumption, each curve in the sequence contains 4 points forming a square, and the sequence of squares can be regarded as (eventually) a sequence in the (sequentially) compact space of the 4-fold product of any closed epsilon enlargement of the area bounded by the original jordan curve. It follows that the sequence of squares contains a convergent subsequence, which can be shown to be a square lying on the original jordan curve.
Thus, proving the C^1 case proves the general case.
This is flawed
The approximation argument is flawed: the squares on approximating curves may have sides decreasing to 0, in which case the limiting "square" degenerates to a point. In fact, Stromquist's theorem covers a much wider class of curves than C^1, but not all continuous curves.
Quantifier
Phrasing should be changed from "Does any..." to "Does every..."

 Drupal
Drupal CSI of Charles University
CSI of Charles University
Already solved
This was proven in https://arxiv.org/pdf/2005.09193.pdf