Home » Subject » Number Theory » Additive N.T.

The Erdos-Turan conjecture on additive bases

Importance: Outstanding ✭✭✭✭
Author(s): Erdos, Paul
Turan, Paul
Subject: Number Theory
» Additive Number Theory
Keywords: additive basis
representation function
Recomm. for undergrads: no
Prize: $500 (Erdos - Graham [EG])
Posted by: mdevos
on: June 8th, 2007

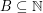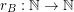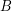Let $ B \subseteq {\mathbb N} $. The representation function $ r_B : {\mathbb N} \rightarrow {\mathbb N} $ for $ B $ is given by the rule 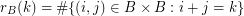$ r_B(k) = \#\{ (i,j) \in B \times B : i + j = k \} $. We call 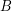$ B $ an additive basis if 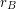$ r_B $ is never $ 0 $.

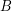Conjecture   If $ B $ is an additive basis, then 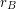$ r_B $ is unbounded.

This famous conjecture seems intuitively likely, but to date, there has been relatively little progress on it despite considerable attention. Two positive results are a theorem of Dirac [D] which shows that 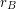$ r_B $ cannot be constant from some point on, and a theorem of Borwein, Choi, and Chu [BCC] which shows that $ r_B $ cannot be bounded above by $ 6 $.

On the other hand, if we consider the related problem for subsets of integers instead of natural numbers, Nathanson [N] has shown that the conjecture does not hold.

Bibliography

[BCC] P. Borwein, S. Choi, and F. Chu, An old conjecture of Erdos-Turan on additive bases, Mathematics of Computation. Volume 75, Number 253, Pages 475–484.

[D] G. A. Dirac, Note on a problem in additive number theory, J. London Math. Soc. 26 (1951), 312–313.

[EG] P. Erdos and R. L. Graham, Old and new problems and results in combinatorial number theory: van der Waerden’s theorem and related topics, Enseign. Math. (2) 25 (1979), no. 3-4, 325–344 (1980). MathSciNet

*[ET] P. Erdos and P. Turan, On a problem of Sidon in additive number theory, and on some related problems, J. London Math. Soc. 16 (1941), 212–215. MathSciNet

[N] Melvyn B. Nathanson, Unique representation bases for the integers, Acta Arith. 108 (2003), no. 1, 1–8. MathSciNet


* indicates original appearance(s) of problem.
add new comment