 login/create account
login/create account
Does every odd number coprime to its Euler totient divide some Carmichael number?
In December 2007 Joe Crump discovered the following Carmichael multiples (of the numbers with previously unknown Carmichael multiples):
- \item
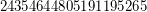 is a Carmichael multiple of
is a Carmichael multiple of  ; \item
; \item 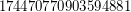 is a Carmichael multiple of
is a Carmichael multiple of 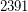 ; \item
; \item 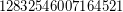 is a Carmichael multiple of
is a Carmichael multiple of 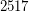 ; \item
; \item 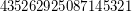 is a Carmichael multiple of
is a Carmichael multiple of 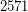 ; \item
; \item 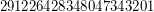 is a Carmichael multiple of
is a Carmichael multiple of 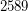 ; \item
; \item 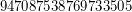 is a Carmichael multiple of
is a Carmichael multiple of 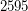 ; \item
; \item 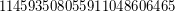 is a Carmichael multiple of
is a Carmichael multiple of 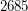 ; \item
; \item 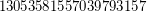 is a Carmichael multiple of
is a Carmichael multiple of 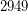 .
. Bibliography
Gérard P. Michon. "Least Carmichael Numbers with Given Divisors (up to 2999)."
Gérard P. Michon and Joseph K. Crump. "Carmichael Multiples of Odd Cyclic Numbers."
W. R. Alford, A. Granville, and C. Pomerance. "There are Infinitely Many Carmichael Numbers." Ann. Math. 139, 703-722, 1994.
Günter Löh and Wolfgang Niebuhr. "A new algorithm for constructing large Carmichael numbers." Math. Comp. 65 (1996), 823-836.
* indicates original appearance(s) of problem.
Утверждение
Утверждение неверно. См. http://dxdy.ru/post577983.html#p577983
From the conjecture's author...
Thanks to Maxal for posting this old conjecture of mine (c. 1980) with a link to the page of computational results which I put online a few years ago. I found out about Maxal's post when someone triggered that link and landed on my own "Numericana" site...
To the best of my knowledge, nobody else has yet worked on this conjecture, so the field is wide open. If you find anything (theoretical or computational) please let me know and I'll post your results, with due credit, at the very location where the statement of the conjecture first appeared, namely at www.numericana.com. Thanks.
Gerard P. Michon, Ph.D.

 Drupal
Drupal CSI of Charles University
CSI of Charles University
2^n == 3 (mod n),
Найдено eще 5 чисел ,удовлятворящию условию 2^n == 3 (mod n), 1. n =2^4700063497-3 2. n =2^3468371109448915-3 3. n =2^8365386194032363-3 4. n =2^10991007971508067-3 5. n =2^63130707451134435989380140059866138830623361447484274774099906755 -3 причем n нечётное, не простое и не делится на 3. Что n нечетное и не делится на 3 выходит из 2^k-3. Что n непростое выходит из 2^k == 3 (mod k), т.е. 2^k -3 = 0 (mod k), т.е. делится на k для выще перечисленных значений. Далее находим следующие 5 чисел где n = 2^(2^k-3)-3 и так далее и так далее до бесконесности. Осюда вытекает следующая Теорема: Чисел удовлятворящию условию 2^n == 3 (mod n) бесконечно много. megamix62@mail.ru (Neymet MS)