A chord of a cycle 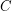is an edge so that 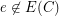, but both ends of are in 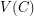. Longest cycles are of great interest in basic graph theory, and this appealing conjecture suggests a very simple property they should share - at least in 3-connected graphs.

In dense graphs, this conjecture is easy to verify - for instance, if is Hamiltonian, it is trivially true. More interestingly, Thomassen [T] proved that his conjecture is true for cubic graphs using a clever sufficient condition for Hamiltonicity (based on Thomason's lollipop method) combined with a pretty theorem of Fleischner and Steibitz (cycle plus triangles graphs are 3-colorable).

Other work on this conjecture has focused on graphs embedded in surfaces. Zhang [Z] has proved the conjecture for planar graphs of minimum degree four, Li and Zhang have proved the conjecture for graphs in the projective plane of minimum degree four [LZ1] and for 4-connected graphs in the klein bottle or torus [LZ2]. Finally, Kawarabayashi, Niu, and Zhang [KNZ] have shown the conjecture for 4-connected graphs on a fixed surface with sufficiently high face-width.