login/create account
Seymour's Second Neighbourhood Conjecture
Conjecture Any oriented graph has a vertex whose outdegree is at most its second outdegree.
By the 
th outdegree of 
, we mean the number of vertices for which the minimal outward-directed path from to them is of length .
Chen, Shen, and Yuster [CSY] proved that in any oriented graph there is a vertex whose second outdegree is at least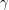 
times its outdegree, where 
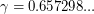is the unique real root of 
.
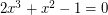This conjecture implies a special case of the \Oprefnum[Caccetta-Häggkvist Conjecture]{46385}.
Bibliography
[ASY] Chen, G.; Shen, J.; Yuster, R. Second neighborhood via first neighborhood in digraphs, Annals of Combinatorics, 7 (2003), 15--20.
[F] Fisher, David C. Squaring a tournament: a proof of Dean's conjecture. J. Graph Theory 23 (1996), no. 1, 43--48.
[KL] Kaneko, Yoshihiro; Locke, Stephen C. The minimum degree approach for Paul Seymour's distance 2 conjecture. Proceedings of the Thirty-second Southeastern International Conference on Combinatorics, Graph Theory and Computing (Baton Rouge, LA, 2001). Congr. Numer. 148 (2001), 201--206.
* indicates original appearance(s) of problem.
Re: It is proved
On December 12th, 2009 Robert Samal says:
Thanks for the reference. The proof, however, seems flawed. The basic outline of the proof (if I understood it correctly) is as follows:
- \item If
has a sink, then this sink satisfies the conditions. \item An oriented graph without directed cycles has a sink. \item Then one proves directly that a graph containing a directed cycle has a vertex (even on that cycle) that satisfies the conditions. The last part (Lemma 2 of the manuscript), is not true -- take a directed cycle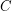 
, add many independent points 
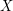, and add all the arcs from 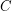to 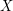. Now the conjecture is true for this graph (one can take any of the sinks -- vertices in 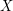), but it is not true that one can choose a vertex of .
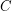The omission in the proof of Lemma 2 is that it's tacitly assumed, that adjacent vertices of the cycle have no common out-neighbours.
So is this proof valid or
On March 14th, 2010 Anonymous says:
So is this proof valid or not? I am currently working on a proof and am wondering whether or not I should be bothering
Re: So is this proof valid or
On August 8th, 2010 rs says:
I believe the mentioned prof is not valid. Is your proof working? And sorry for the late reply, our system falsely recognized your comment as a spam.
Counterexample for the proof
On March 18th, 2011 sjcjoosten says:
As was indicated, Lemma 2 is false. Here is a counterexample to lemma 2. Note that it is not a counterexample to the conjecture, since it has two sinks.
Drupal
CSI of Charles University
It is proved
This conjecture has been proved. You can find the proof here .