 login/create account
login/create account
Double-critical graph conjecture
A connected simple graph  is called double-critical, if removing any pair of adjacent vertexes lowers the chromatic number by two.
is called double-critical, if removing any pair of adjacent vertexes lowers the chromatic number by two.
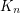 is the only
is the only  -chromatic double-critical graph
-chromatic double-critical graph
This conjecture is a special case of a more general problem by Erdos and Lovasz proposed in 1966. It has been independently proven for the case where 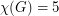 by Mozhan [3] and Stiebitz [4].
by Mozhan [3] and Stiebitz [4].
Bibliography
*[1] P. Erdos, Problem 2, in: Theory of Graphs (Proc. Colloq., Tihany, 1966), Academic Press, New York, 1968, p. 361.
[2] F. Chung, R. Graham, Erdos on graphs: His legacy of unsolved problems, A K Peters, Wellesley, Massachusetts, 1998.
[3] N. N. Mozhan, On double critical graphs with the chromatic number five, Metody Diskretb. Anal. 46 (1987) 50-59.
[4] M. Stiebitz, 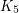 is the only double-critical
is the only double-critical  -chromatic graph, Discrete Math. 64 (1987) 91-93.
-chromatic graph, Discrete Math. 64 (1987) 91-93.
* indicates original appearance(s) of problem.
missing connectivity condition
As it is now the conjecture is false -- take the disjoint union of a clique and a vertex. Just need to add "connected" to the definition of doubly critical.

 Drupal
Drupal CSI of Charles University
CSI of Charles University
Statement needs connected assumption
G needs to be assumed connected in the statement. Otherwise the disjoint union of K_k and a vertex (say) would be a counterexample.