 login/create account
login/create account
choosability
Choosability of Graph Powers ★★
Author(s): Noel
 such that for every graph
such that for every graph  ,
, ![\[\text{ch}\left(G^2\right)\leq f\left(\chi\left(G^2\right)\right)?\]](/files/tex/989db06683633e86605c26e7d9f0bffc7e46a496.png)
Keywords: choosability; chromatic number; list coloring; square of a graph
Bounding the on-line choice number in terms of the choice number ★★
Author(s): Zhu
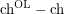 is arbitrarily large?
is arbitrarily large? Keywords: choosability; list coloring; on-line choosability
Choice number of complete multipartite graphs with parts of size 4 ★
Author(s):
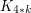 for general
for general  ?
? Keywords: choosability; complete multipartite graph; list coloring
Choice Number of k-Chromatic Graphs of Bounded Order ★★
Author(s): Noel
 is a
is a  -chromatic graph on at most
-chromatic graph on at most 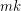 vertices, then
vertices, then 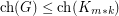 .
. Keywords: choosability; complete multipartite graph; list coloring
Circular choosability of planar graphs ★
Author(s): Mohar
Let 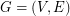 be a graph. If
be a graph. If  and
and 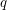 are two integers, a
are two integers, a 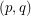 -colouring of
-colouring of  is a function
is a function  from
from 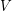 to
to 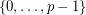 such that
such that 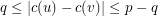 for each edge
for each edge 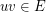 . Given a list assignment
. Given a list assignment  of
of  , i.e.~a mapping that assigns to every vertex
, i.e.~a mapping that assigns to every vertex  a set of non-negative integers, an
a set of non-negative integers, an  -colouring of
-colouring of  is a mapping
is a mapping 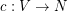 such that
such that 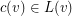 for every
for every 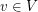 . A list assignment
. A list assignment  is a
is a  -
-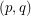 -list-assignment if
-list-assignment if 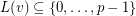 and
and 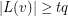 for each vertex
for each vertex 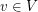 . Given such a list assignment
. Given such a list assignment  , the graph G is
, the graph G is 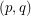 -
- -colourable if there exists a
-colourable if there exists a 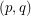 -
- -colouring
-colouring  , i.e.
, i.e.  is both a
is both a 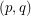 -colouring and an
-colouring and an  -colouring. For any real number
-colouring. For any real number 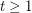 , the graph
, the graph  is
is  -
-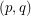 -choosable if it is
-choosable if it is 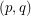 -
- -colourable for every
-colourable for every  -
-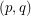 -list-assignment
-list-assignment  . Last,
. Last,  is circularly
is circularly  -choosable if it is
-choosable if it is  -
-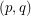 -choosable for any
-choosable for any  ,
, 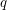 . The circular choosability (or circular list chromatic number or circular choice number) of G is
. The circular choosability (or circular list chromatic number or circular choice number) of G is 
Keywords: choosability; circular colouring; planar graphs
Ohba's Conjecture ★★
Author(s): Ohba
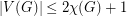 , then
, then  .
. Keywords: choosability; chromatic number; complete multipartite graph; list coloring

 Drupal
Drupal CSI of Charles University
CSI of Charles University